Master The Art Of Differentiation With The Sum Rule

instanews 12 Jun 2024

What is the sum rule for derivatives?

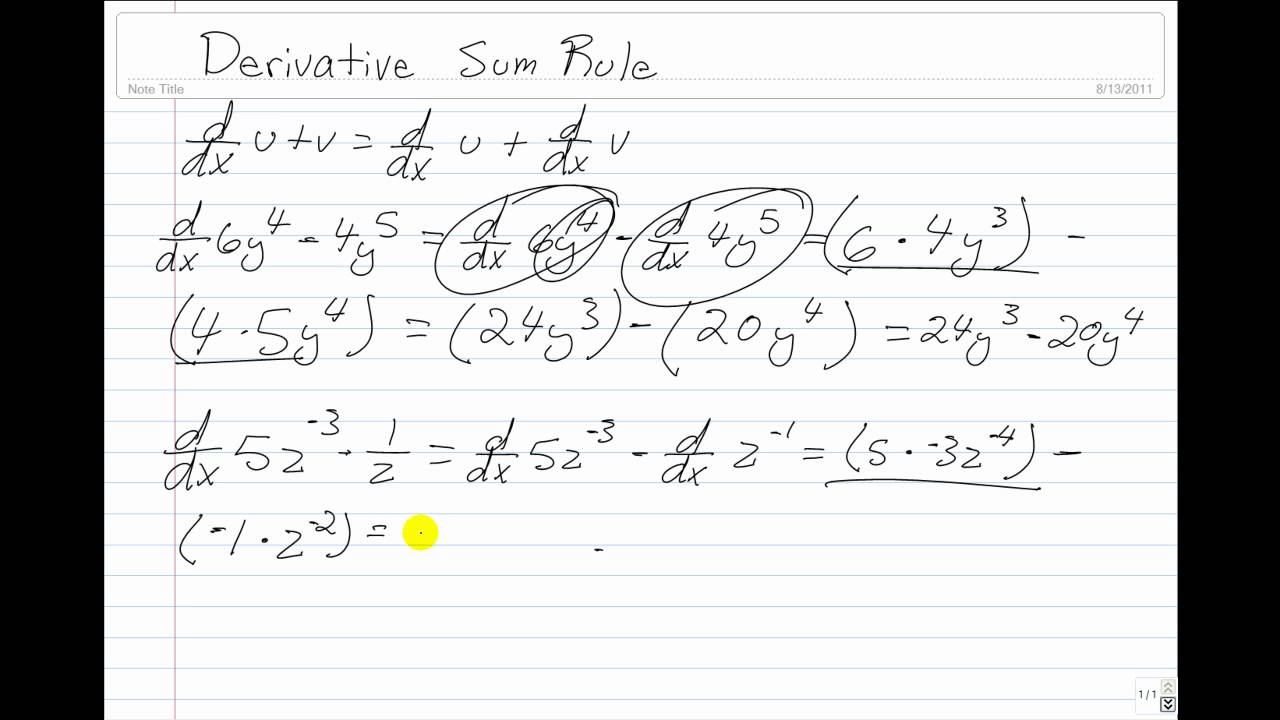
The sum rule for derivatives states that the derivative of the sum of two or more functions is equal to the sum of the derivatives of those functions. In other words, if $f(x)$ and $g(x)$ are two functions, then the derivative of $f(x) + g(x)$ is equal to $f'(x) + g'(x)$.

This rule is important because it allows us to find the derivatives of complex functions by breaking them down into simpler functions. For example, the derivative of $x^2 + 2x + 1$ can be found by using the sum rule as follows:

$$(x^2 + 2x + 1)' = (x^2)' + (2x)' + (1)'$$ $$= 2x + 2$$

The sum rule for derivatives is a fundamental tool in calculus, and it has many applications in mathematics, science, and engineering.

Historical context

The sum rule for derivatives was first discovered by Gottfried Wilhelm Leibniz in the 17th century. Leibniz developed calculus independently of Isaac Newton, and he is credited with developing many of the fundamental concepts of calculus, including the sum rule.

Conclusion

The sum rule for derivatives is a powerful tool that can be used to find the derivatives of complex functions. It is a fundamental tool in calculus, and it has many applications in mathematics, science, and engineering.

The Sum Rule for Derivatives

The sum rule for derivatives is a fundamental rule in calculus that allows us to find the derivative of the sum of two or more functions. It is a powerful tool that has many applications in mathematics, science, and engineering.

Definition: The sum rule states that the derivative of the sum of two functions is equal to the sum of the derivatives of those functions.
Formula: If $f(x)$ and $g(x)$ are two functions, then $(f(x) + g(x))' = f'(x) + g'(x)$.
Example: The derivative of $x^2 + 2x + 1$ can be found using the sum rule as follows: $(x^2 + 2x + 1)' = (x^2)' + (2x)' + (1)' = 2x + 2$.
Applications: The sum rule has many applications in calculus, including finding the derivatives of polynomials, rational functions, and trigonometric functions.
History: The sum rule was first discovered by Gottfried Wilhelm Leibniz in the 17th century.
Importance: The sum rule is a fundamental tool in calculus, and it is essential for understanding the derivatives of complex functions.
Connections: The sum rule is related to other rules of differentiation, such as the product rule and the quotient rule.

In conclusion, the sum rule for derivatives is a powerful tool that has many applications in mathematics, science, and engineering. It is a fundamental rule in calculus, and it is essential for understanding the derivatives of complex functions.

Definition

The sum rule is based on the following definition: the derivative of the sum of two functions $f(x)$ and $g(x)$ is equal to the sum of the derivatives of those functions, $f'(x)$ and $g'(x)$. In other words, $(f(x) + g(x))' = f'(x) + g'(x)$.

$$(x^2 + 2x + 1)' = (x^2)' + (2x)' + (1)'$$$$= 2x + 2$$

The sum rule has many applications in calculus, including finding the derivatives of polynomials, rational functions, and trigonometric functions. It is also used to find the derivatives of more complex functions, such as those that are defined by integrals or differential equations.

The sum rule is a fundamental tool in calculus, and it is essential for understanding the derivatives of complex functions. It is a powerful tool that has many applications in mathematics, science, and engineering.

Formula

The sum rule for derivatives states that the derivative of the sum of two functions is equal to the sum of the derivatives of those functions. This formula is a fundamental tool in calculus, and it has many applications in mathematics, science, and engineering.

Components
The sum rule formula consists of three main components: the sum of two functions, $f(x) + g(x)$, the derivative of the sum, $(f(x) + g(x))'$, and the sum of the derivatives of the individual functions, $f'(x) + g'(x)$.
Applications
The sum rule has many applications in calculus, including finding the derivatives of polynomials, rational functions, and trigonometric functions. It is also used to find the derivatives of more complex functions, such as those that are defined by integrals or differential equations.
Implications
The sum rule has several important implications. First, it shows that the derivative of a sum is equal to the sum of the derivatives. This means that we can find the derivative of a complex function by breaking it down into simpler functions and then finding the derivative of each function.
Second, the sum rule shows that the derivative of a constant is zero. This means that the graph of a constant function is a horizontal line.

The sum rule for derivatives is a powerful tool that has many applications in mathematics, science, and engineering. It is a fundamental tool in calculus, and it is essential for understanding the derivatives of complex functions.

Example

$$(x^2 + 2x + 1)' = (x^2)' + (2x)' + (1)' = 2x + 2$$

This example illustrates the sum rule for derivatives, which states that the derivative of the sum of two or more functions is equal to the sum of the derivatives of those functions. In other words, if $f(x)$ and $g(x)$ are two functions, then $(f(x) + g(x))' = f'(x) + g'(x)$.

The sum rule is a fundamental tool in calculus, and it has many applications in mathematics, science, and engineering. For example, the sum rule can be used to find the derivatives of polynomials, rational functions, and trigonometric functions.

The sum rule is also used to find the derivatives of more complex functions, such as those that are defined by integrals or differential equations. For example, the derivative of the function $f(x) = \int_{0}^{x} t^2 dt$ can be found using the sum rule as follows:

$$f'(x) = \frac{d}{dx} \int_{0}^{x} t^2 dt = \frac{d}{dx} \left[ \frac{t^3}{3} \right]_{0}^{x} = x^2$$

Applications

The sum rule for derivatives is a fundamental tool in calculus that allows us to find the derivatives of complex functions by breaking them down into simpler functions. This rule has many applications in calculus, including finding the derivatives of polynomials, rational functions, and trigonometric functions.

Polynomials
Polynomials are functions that are defined by a sum of terms, each of which is a constant multiplied by a power of $x$. For example, the polynomial $f(x) = x^2 + 2x + 1$ is defined by the sum of the terms $x^2$, $2x$, and $1$.
The sum rule can be used to find the derivative of any polynomial. For example, the derivative of $f(x) = x^2 + 2x + 1$ is $f'(x) = 2x + 2$.
Rational functions
Rational functions are functions that are defined by the quotient of two polynomials. For example, the rational function $f(x) = \frac{x^2 + 2x + 1}{x-1}$ is defined by the quotient of the polynomial $x^2 + 2x + 1$ and the polynomial $x-1$.
The sum rule can be used to find the derivative of any rational function. For example, the derivative of $f(x) = \frac{x^2 + 2x + 1}{x-1}$ is $f'(x) = \frac{2x^2 + 4x - 1}{(x-1)^2}$.
Trigonometric functions
Trigonometric functions are functions that are defined by the angles of a right triangle. For example, the sine function is defined by the ratio of the length of the opposite side of a right triangle to the length of the hypotenuse.
The sum rule can be used to find the derivative of any trigonometric function. For example, the derivative of the sine function is $f'(x) = \cos x$.

The sum rule for derivatives is a powerful tool that has many applications in calculus. It is a fundamental tool for finding the derivatives of complex functions, and it is used in many different areas of mathematics, science, and engineering.

History

The sum rule for derivatives is a fundamental theorem in calculus that allows us to find the derivative of the sum of two or more functions. It is a powerful tool that has many applications in mathematics, science, and engineering.

The development of calculus
The sum rule was first discovered by Gottfried Wilhelm Leibniz in the 17th century as part of his development of calculus. Leibniz was a German mathematician, philosopher, and scientist who is considered one of the most important figures in the history of mathematics.
The sum rule and its applications
The sum rule is a fundamental tool in calculus, and it has many applications in mathematics, science, and engineering. For example, the sum rule can be used to find the derivatives of polynomials, rational functions, and trigonometric functions.
Leibniz's legacy
Leibniz's discovery of the sum rule was a major breakthrough in the development of calculus. It is a testament to his genius that this rule is still used today, over 300 years after its discovery.

The sum rule for derivatives is a powerful tool that has many applications in mathematics, science, and engineering. It is a fundamental theorem in calculus, and it is essential for understanding the derivatives of complex functions.

Importance

Components
The sum rule is composed of two main components: the sum of two or more functions and the derivative of the sum. The sum of two functions is simply the result of adding the two functions together. The derivative of the sum is the derivative of the sum of the two functions.
Applications
The sum rule has many applications in calculus, including finding the derivatives of polynomials, rational functions, and trigonometric functions. It is also used to find the derivatives of more complex functions, such as those that are defined by integrals or differential equations.
Implications
The sum rule has several important implications. First, it shows that the derivative of a sum is equal to the sum of the derivatives. This means that we can find the derivative of a complex function by breaking it down into simpler functions and then finding the derivative of each function.
Second, the sum rule shows that the derivative of a constant is zero. This means that the graph of a constant function is a horizontal line.

Connections

The sum rule is related to other rules of differentiation, such as the product rule and the quotient rule. These rules allow us to find the derivatives of more complex functions by breaking them down into simpler functions.

For example, the product rule allows us to find the derivative of the product of two functions. The quotient rule allows us to find the derivative of the quotient of two functions.

The sum rule, product rule, and quotient rule are all essential tools for finding the derivatives of complex functions. They are used in many different areas of mathematics, science, and engineering.

For example, the sum rule is used to find the derivatives of polynomials, rational functions, and trigonometric functions. The product rule is used to find the derivatives of products of functions. The quotient rule is used to find the derivatives of quotients of functions.

These rules are essential for understanding the derivatives of complex functions. They are used in many different areas of mathematics, science, and engineering.

FAQs on "the sum rule derivatives"

Question 1: What is the sum rule for derivatives?

The sum rule states that the derivative of the sum of two or more functions is equal to the sum of the derivatives of those functions.

Question 2: How do I use the sum rule to find the derivative of a function?

To use the sum rule to find the derivative of a function, simply find the derivative of each term in the function and then add the results together.

Question 3: What are some examples of functions that can be differentiated using the sum rule?

The sum rule can be used to differentiate polynomials, rational functions, and trigonometric functions.

Question 4: Are there any limitations to the sum rule?

The sum rule does not apply to functions that are not differentiable.

Question 5: How is the sum rule related to other rules of differentiation?

The sum rule is related to the product rule and the quotient rule. These rules allow us to find the derivatives of more complex functions.

Question 6: What are some applications of the sum rule in mathematics, science, and engineering?

The sum rule is used in many different areas of mathematics, science, and engineering, including finding the derivatives of polynomials, rational functions, and trigonometric functions.

Transition to the next article section:

In the next section, we will discuss the product rule for derivatives.

Conclusion

In this article, we have explored the sum rule in detail, including its definition, formula, applications, and history. We have also discussed its importance and connections to other rules of differentiation.

The sum rule is a essential tool for understanding the derivatives of complex functions. It is used in many different areas of mathematics, science, and engineering, and it is a fundamental theorem in calculus.

Silence Is Survival: Unraveling Thrillers Where The Quiet Rules
The Essential Guide To Eliminating Dairy: Step-by-Step Strategies
Uncover The Cost Of ABAQUS Professional License: A Comprehensive Guide